DC Motor Speed: Voltage and Torque Relationships

Something we have discussed in the past is how varying the voltage to a vibration motor can change the amplitude, particularly with reference to haptic feedback and playing different effects. However, something we haven’t explicitly shown is why this happens, or discussed it in the context of a normal DC motor. So in this short article, we will demonstrate how an increased voltage will increase the speed and an increased load will decrease the speed of a motor.

It is important to note that this is valid for all of our products that are based on brushed DC motors, so it applies to:

• DC motors, directly
• DC gearmotors, directly with a simple mechanical speed reduction
• Vibration motors, which have a fixed load

We start with the equivalent circuit of a brushed DC motor:

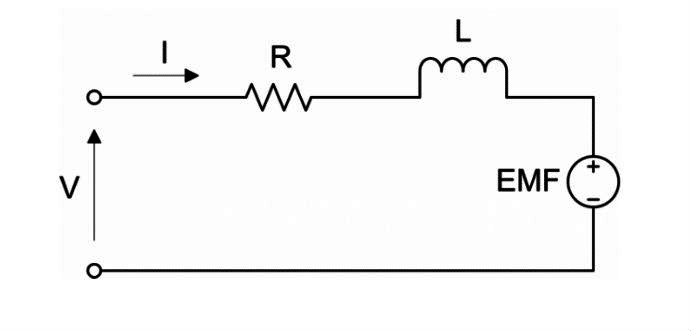
Using a simple voltage loop we can see that the input voltage (\(V)\) is equal to the voltage drop across the coil resistance (\(R)\) and the inductor (\(L)\), with the back EMF (\(E)\) produced by the motor in motion, i.e.:

$$V = IR + L \frac{dI}{dt} + E$$

However, to demonstrate the effect voltage and torque have on speed, we are only concerned with steady-state behaviour at the moment. In steady-state we benefit from two simplifications:

• First, the current is constant which means the inductor \( L\) can be removed
• Secondly, as there is no change in speed, the torque produced by the motor and the torque produced by the load must be equal

The second simplification helps as we consider how torque is produced in the motor, given by the following equation:

$$T = K_{T} \phi I$$

Where \(K_{T}\) represents a constant inherited from its internal design, \(\phi\) is the total flux and \(T\) can represent our load torque. We rearrange this for \(I\) and enter it into our original equation:

$$V = \frac{T}{K_{T} \phi} R + E$$

Now we turn our attention to the emf \(E\), which is dependent upon the total flux \(\phi\) motor specific factors (such as its radius and length) that make a second constant \(K_{E}\) and the speed of the motor \(n\):

$$E = K_{E} \phi n$$

Substituting this value of \(E\) into our equation yields the following:

$$V = \frac{T}{K_{T} \phi} R + K_{E} \phi n$$

The equation above actually represents a linear motor, in adapting this to an angular rotating motor we consider the flux to be constant at its full value. In doing so it is combined with each constant to produce the torque constant and electrical constant of the motor, denoted \(k_{t}\) and \(k_{e}\) respectively. As discussed before, these constants actually share the same units, so we can replace both constants in our equation to the value \(k\). Also, we now replace linear speed \(n\) with angular velocity \(\omega\), so our equation becomes:

$$V = \frac{T}{k} R + k \omega$$

Rearranged for angular velocity:

$$\omega = \frac{V}{k} – \frac {T}{k^{2}} R$$

In summary, we can highlight the two main variables which affect the speed of the motor in our final equation:

1. Input Voltage:  For a fixed load, the speed of the motor is affected by the applied voltage. Increase in voltage = increase in speed
2. Load Torque: For a fixed voltage, the speed of the motor is inversely affected by the load. Increase in load torque = decrease in speed

If you have any questions about this relationship, or how the theory applies to a ‘real-world’ motor, please contact our sales engineers to help with your application.

---